Sudoku: Beginners Guide to Solving Puzzles

Sudoku puzzles can be both challenging and rewarding. If you’re new to Sudoku and want to learn how to solve these puzzles, you’ve come to the right place. In this beginner’s guide, we’ll walk you through the steps to successfully tackle Sudoku grids. Let’s get started!

Understanding the Rules

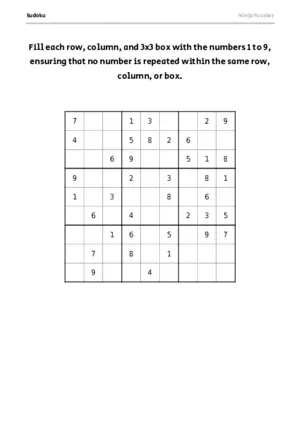
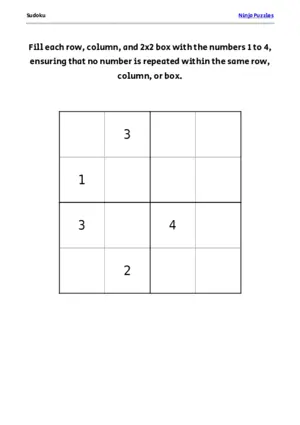
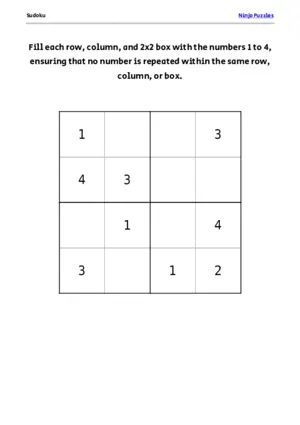
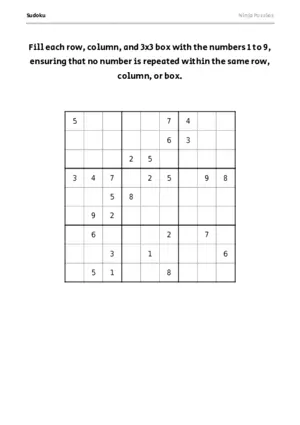
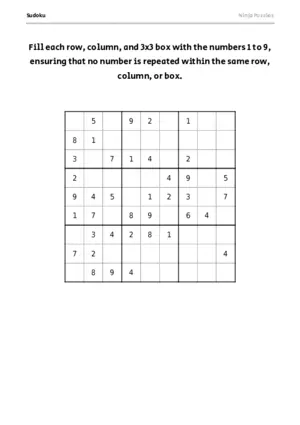
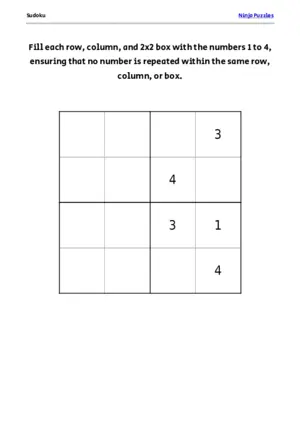
Sudoku is played on a 9x9 grid, which is further divided into nine 3x3 subgrids. The objective is to fill in the grid so that each row, column, and subgrid contains the numbers 1 to 9 without repetition. Remember these rules as they form the foundation for solving Sudoku puzzles.

Step-by-Step Solving Process

Start with the easy clues: Begin by identifying the numbers already provided in the puzzle. These are usually the easiest clues to find. Fill in the given numbers in their respective cells.
Work on rows, columns, and subgrids: Analyze each row, column, and subgrid individually. Look for missing numbers and try to determine their correct placement. Begin by identifying the numbers that can only fit in one specific cell within a row, column, or subgrid. Fill in these numbers.
Use elimination: For each empty cell, consider the numbers already present in the same row, column, and subgrid. Eliminate the numbers that are already present. If only one possibility remains for a particular cell, fill it in.
Repeat the process of elimination: As you fill in numbers, you’ll discover new possibilities and eliminate certain numbers from other cells. Keep repeating the elimination process until no more numbers can be filled using this method.
Look for number patterns: Observe any emerging patterns within the puzzle. For instance, if you find two cells in a row, column, or subgrid that can only contain the same two numbers, eliminate those numbers from the possibilities in other cells within that area.
Use the “what-if” technique: If you reach a point where no further progress can be made, consider guessing a number for a particular cell that seems most likely and continue solving based on that guess. If you encounter a contradiction, backtrack and try another number until the puzzle is complete.
Practice regularly: Improving your Sudoku-solving skills comes with practice. Start with easier puzzles and gradually move on to more difficult ones as you gain confidence and experience.

Remember, Sudoku puzzles rely on logical reasoning and deduction rather than guesswork. By following these steps and practicing regularly, you’ll become more proficient at solving Sudoku puzzles. Enjoy the process and have fun!

We hope this beginner’s guide has been helpful to you. Happy Sudoku solving!

Sudoku Solver

If you get really stuck then we have an online Sudoku Solver, and a Sudoku Mini Solver to help you solve the most challenging Sudoku puzzles.

Advanced Sudoku Strategies

Singleton Technique: This strategy involves identifying squares that have only one possible candidate number based on the numbers already present in the row, column, and box.
Naked Pairs and Triples: When two or three squares in a unit (row, column, or box) have the same two or three candidate numbers, those numbers can be eliminated as possibilities from other squares in the unit.
Hidden Pairs and Triples: This strategy focuses on identifying two or three candidate numbers that only appear in the same two or three squares within a unit. These numbers can then be eliminated as possibilities from other squares in the unit.
Sudoku X-Wing: Sudoku X-Wing involves finding two rows or two columns where the same number can only occur in the same two positions. By eliminating that number as a possibility in the corresponding rows or columns, you can make further deductions.
Swordfish: Similar to Sudoku X-Wing, Swordfish strategy expands the concept to three rows or three columns. It involves finding three rows or three columns where the same number can only occur in the same three positions, allowing for further eliminations.
Sudoku Y-Wing: Sudoku Y-Wing is an advanced strategy that involves three cells and three numbers. By utilizing a specific pattern of candidate numbers, you can make deductions that lead to solving other squares.
Sudoku XYZ-Wing: XYZ-Wing involves three cells and three numbers, with each number belonging to a different candidate list. It allows for deductions by creating chains of eliminations based on the relationships between the three numbers.
Coloring: This strategy involves assigning colors (usually two) to candidates and using the relationships between these colors to make deductions.
Backtracking: Backtracking is a brute-force technique that involves making educated guesses and trying different possibilities until a contradiction is reached or the puzzle is solved.